Rational numbers

A number that appears as a ratio of any two integers is called a rational number.

Introduction

The integers are often appeared in antecedent and consequent positions of the ratio in some cases. The ratio of them is also a number and it is called as a rational number.

Examples

$\dfrac{1}{4}$, $\dfrac{-7}{2}$, $\dfrac{0}{8}$, $\dfrac{11}{8}$, $\dfrac{15}{5}$, $\dfrac{14}{-7}$, $\cdots$

Usage

The rational numbers are mainly used to represent the fractions in mathematical form.

Rules of formation

There are two rules for forming the rational numbers by the integers.

1. The antecedent can be any integer.
2. The consequent should be a non-zero integer.

Note

$10$ and $2$ are two integers and find the ratio of $10$ to $2$ by the division.

$Ratio \,=\, \dfrac{10}{2}$

It is a rational number basically and now, find their quotient.

$\implies$ $\dfrac{10}{2}$ $\,=\,$ $5$

It proves that a rational number can be an integer but an integer may not always be a rational number.

Formation

The heights of a boy and his sister are $150 \, cm$ and $100 \, cm$ respectively.

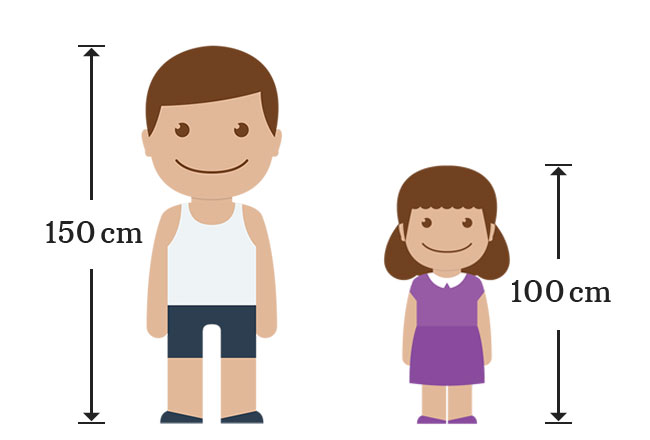
Calculate the ratio of boy’s height to his sister’s height.

$Ratio \,=\, \dfrac{150}{100}$
$\implies$ $\require{cancel} Ratio \,=\, \dfrac{\cancel{150}}{\cancel{100}}$
$\implies$ $\require{cancel} Ratio \,=\, \dfrac{3}{2}$

Similarly, calculate the ratio of girl’s height to her brother’s height.

$Ratio \,=\, \dfrac{100}{150}$
$\implies$ $\require{cancel} Ratio \,=\, \dfrac{\cancel{100}}{\cancel{150}}$
$\implies$ $\require{cancel} Ratio \,=\, \dfrac{2}{3}$

$\dfrac{2}{3}$ and $\dfrac{3}{2}$ are two ratios but $2$ and $3$ are integers. So, if any two integers are expressed in ratio form, then they are called the rational numbers. Therefore, $\dfrac{2}{3}$ and $\dfrac{3}{2}$ are called as the rational numbers.

Representation

The collection of all rational numbers can be represented as a set and denoted by $Q$, which is a first letter of the “Quotient”. The rational numbers are infinite. So, the set of rational numbers is called as an infinite set.

$Q$ $\,=\,$ $\Big\{\cdots, -2, \dfrac{-9}{7}, -1, \dfrac{-1}{2}, 0, \dfrac{3}{4}, 1, \dfrac{7}{6}, 2, \cdots\Big\}$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.