How to solve the Equal squares equation

Formula

$x \,=\, \pm \, a,$ if the $x^2 \,=\, a^2$

Introduction

The quantities in square form are involved in the equations in some cases. In a special case, the square of a quantity is equal to the square of another quantity. Now, let’s learn how to solve the equation in which the squares of two quantities are equal.

1. Let’s denote a variable by $x$ and its square is written as $x^2$.
2. Similarly, let’s represent a constant by $a$ and its square is written as $a^2$.

Let’s consider that the square of variable $x$ is equal to the square of constant $a$, and it is expressed in mathematics as follows.

$x^2 \,=\, a^2$

Solution

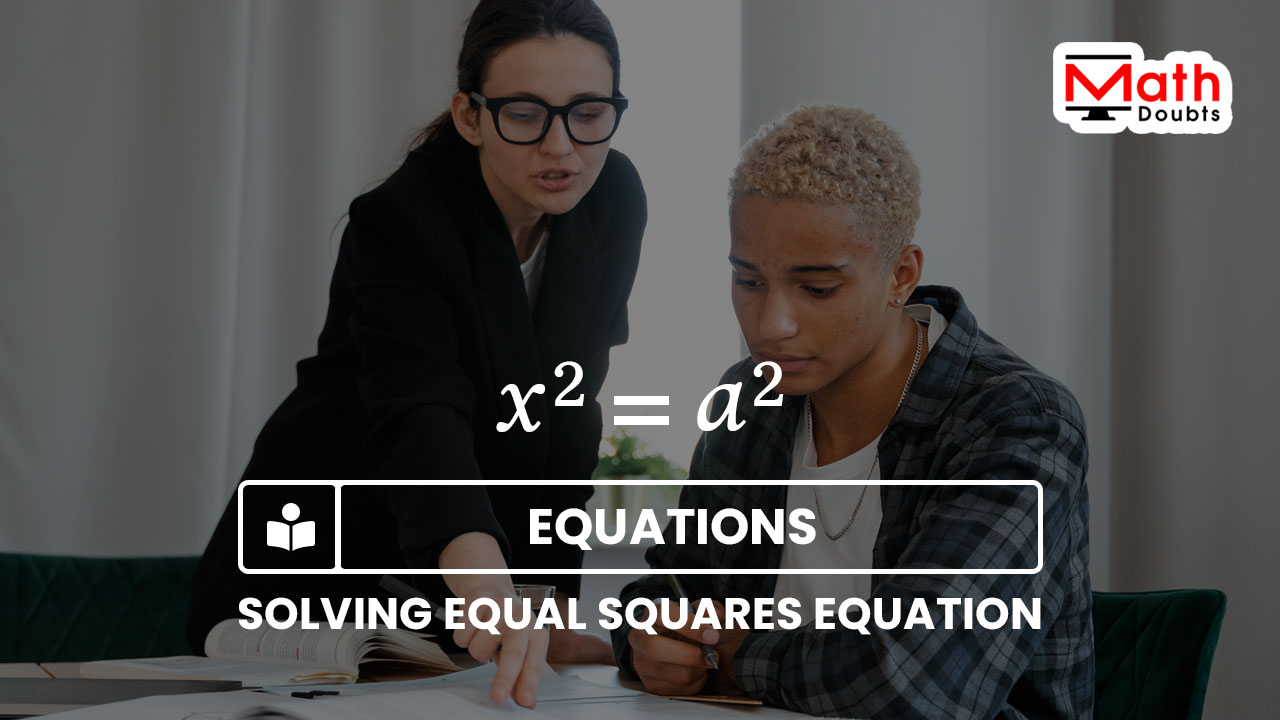
It is time to learn how to solve this equation in mathematics. Firstly, subtract both sides of expressions by the square of $a$, to make the expression on the right-hand side of equation equals to zero.

$\implies$ $x^2-a^2$ $\,=\,$ $a^2-a^2$

$\implies$ $x^2-a^2$ $\,=\,$ $\cancel{a^2}-\cancel{a^2}$

$\,\,\,\therefore\,\,\,\,\,\,$ $x^2-a^2 \,=\, 0$

On the left-hand side of the equation, the expression represents the difference of squares, and it is also a quadratic equation. The equation that contains the quantities in square form can be solved by using the difference of squares formula.

The equation involving the quantities in square form can be factored now as a product of two linear expressions in one variable by using the difference of squares identity.

$\implies$ $(x+a)(x-a) \,=\, 0$

It is time to solve the equation. In this case, either the binomial $x+a$ or $x-a$ is equal to zero.

$\implies$ $x+a \,=\, 0$ or $x-a \,=\, 0$

Now, let’s find the value of $x$ by solving each linear equation in one variable.

$\,\,\,\therefore\,\,\,\,\,\,$ $x \,=\, -a$ or $x \,=\, a$

Therefore, it is solved that the value of $x$ is equal to negative $a$ or positive $a$. It is simply written in mathematical form as follows.

$\,\,\,\therefore\,\,\,\,\,\,$ $x \,=\, \pm \, a$

Therefore, it is proved that the value of a variable is equal to the plus or minus constant when the square of a variable is equal to the square of a constant.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.