The distributive property of multiplication over addition can be proved in algebraic form by the geometrical approach. It is actually derived in mathematics by the area of a rectangle.

You can observe that the widths of both rectangles are same.
The length and width of first rectangle are $b$ and $a$ respectively. Now, find the area of this rectangle.
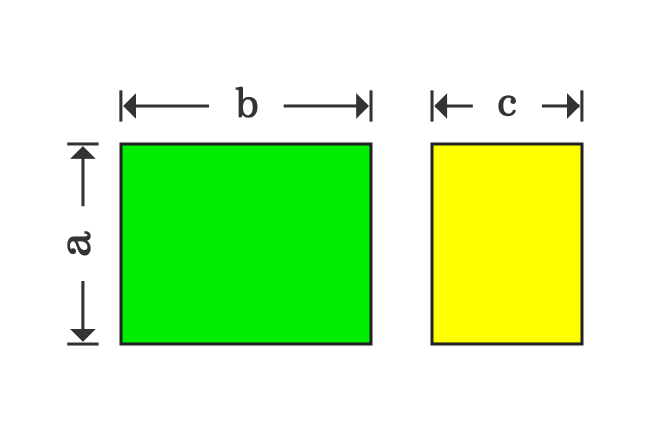
$Area \,=\, b \times a$
$\implies$ $Area \,=\, a \times b$
$\,\,\, \therefore \,\,\,\,\,\,$ $Area \,=\, ab$
The length and width of second rectangle are $c$ and $a$ respectively and find the area of this rectangle.
$Area \,=\, c \times a$
$\implies$ $Area \,=\, a \times c$
$\,\,\, \therefore \,\,\,\,\,\,$ $Area \,=\, ac$
Now, find the sum of the areas of the two rectangles.
$\,\,\, \therefore \,\,\,\,\,\,$ $Area \,=\, ab+ac$
Now, join both rectangles to get the actual rectangle. The lengths of both rectangles are $b$ and $c$ respectively and their sum is equal to the length of the actual rectangle.
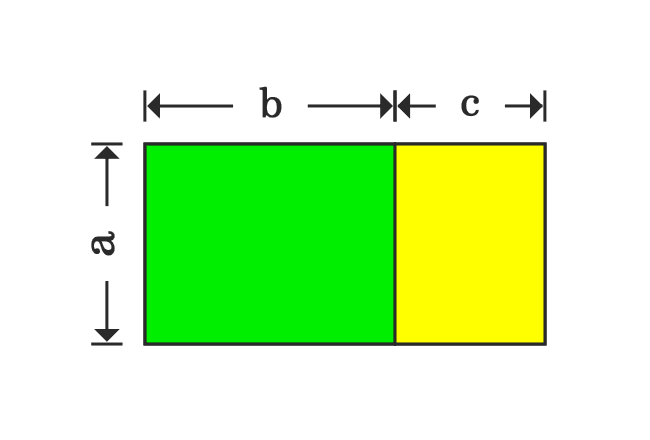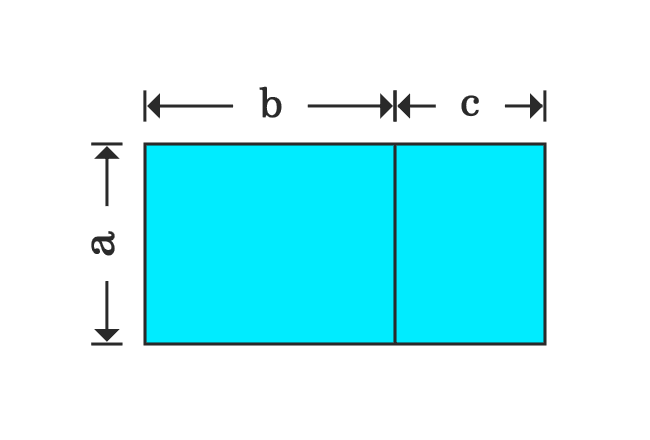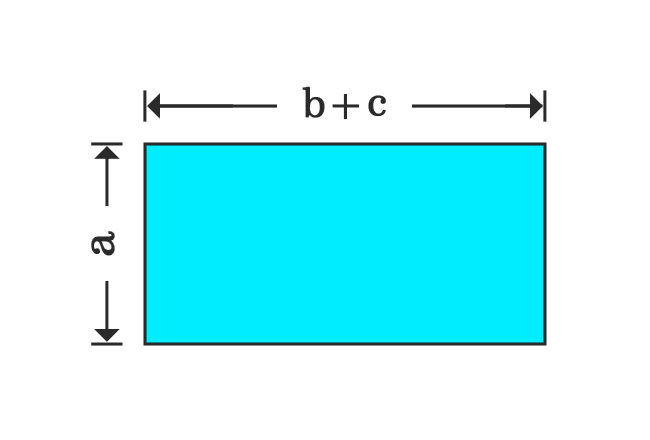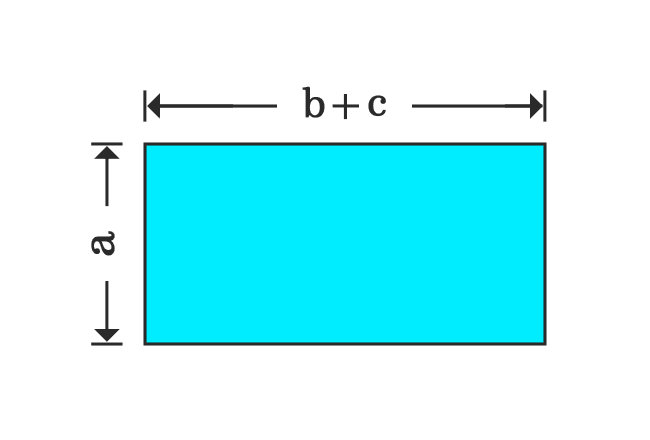
Therefore, the length and width of the rectangle are $b+c$ and $a$ respectively and find the area of this rectangle.
$Area \,=\, (b+c) \times a$
$\implies$ $Area \,=\, a \times (b+c)$
$\,\,\, \therefore \,\,\,\,\,\,$ $Area \,=\, a(b+c)$
The area of the rectangle is $a(b+c)$ and it is divided as two rectangles. So, the area of the rectangle is equal to the sum of the areas of the two small rectangles.
$\therefore \,\,\,\,\,\,$ $a(b+c) \,=\, ab+ac$
A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.
Copyright © 2012 - 2023 Math Doubts, All Rights Reserved