Solve the Quadratic equation $x^2$ $-$ $4x$ $+$ $4$ $\,=\,$ $0$ by Quadratic formula

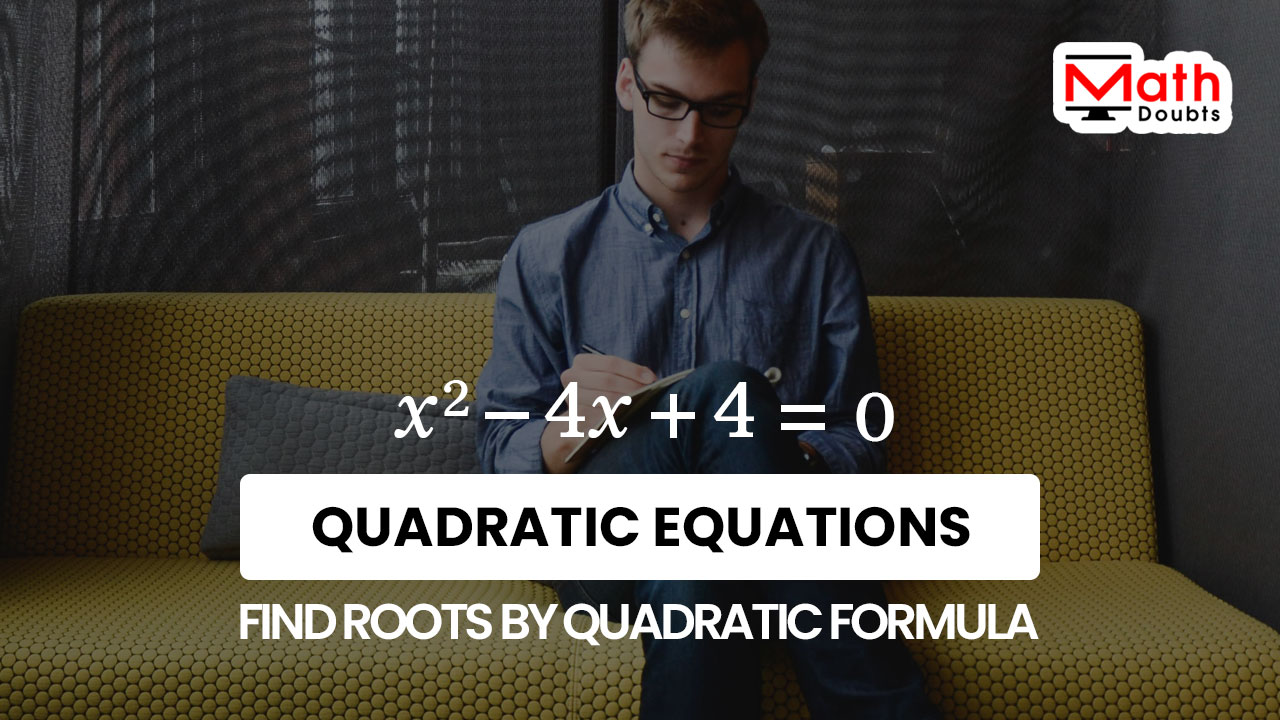
The square of $x$ minus four times $x$ plus four equals to zero is a quadratic equation and it should be calculated by the quadratic formula in this quadratic equation problem. Let us learn how to find the zeros of a quadratic equation $x^2-4x+4$ $\,=\,$ $0$ by the quadratic formula.

Compare the equation with standard form

Firstly, compare the quadratic equation $x^2-4x+4$ $\,=\,$ $0$ with the standard form of a quadratic equation $ax^2+bx+c$ $\,=\,$ $0$ to find the values of $a$, $b$ and $c$.

1. $a \,=\, 1$
2. $b \,=\, -4$
3. $c \,=\, 4$

Substitute the values in Quadratic formula

Now, substitute $a = 1$, $b = -4$ and $c = 4$ in the quadratic formula to find the roots of a quadratic equation $x$ square minus $4x$ plus $4$ equals to zero.

$\implies$ $x$ $\,=\,$ $\dfrac{-(-4)\pm \sqrt{(-4)^2-4 \times 1 \times 4}}{2 \times 1}$

Find the roots of equation by simplification

The value of $x$ is expressed as a fraction in arithmetic form. It is time to find the values of $x$ by simplifying the arithmetic expression on the right hand side of the equation.

$=\,\,$ $\dfrac{4\pm\sqrt{16-4 \times 4}}{2}$

$=\,\,$ $\dfrac{4\pm\sqrt{16-16}}{2}$

$=\,\,$ $\dfrac{4\pm\sqrt{0}}{2}$

$=\,\,$ $\dfrac{4\pm 0}{2}$

$=\,\,$ $\dfrac{4}{2}$

$=\,\,$ $\dfrac{\cancel{4}}{\cancel{2}}$

$\,\,\,\therefore\,\,\,\,\,\,$ $x \,=\, 2$

The quadratic equation $x^2-4x+4 \,=\, 0$ is solved by the quadratic formula. A quadratic equation should have two roots but it is evaluated that $x = 2$, which is only one root.

It clears that the two roots of a quadratic equation $x^2-4x+4 \,=\, 0$ are real but equal. Therefore, the solution set with repeated zeros is written as $\{2, 2\}$ in mathematics.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.