Evaluate $\displaystyle \int{\dfrac{1}{\sqrt{x^2+x+1}}}\,dx$

The indefinite integral of the reciprocal of the square root of $x$ square plus $x$ plus one with respect to $x$ should have to evaluate in this integration problem.

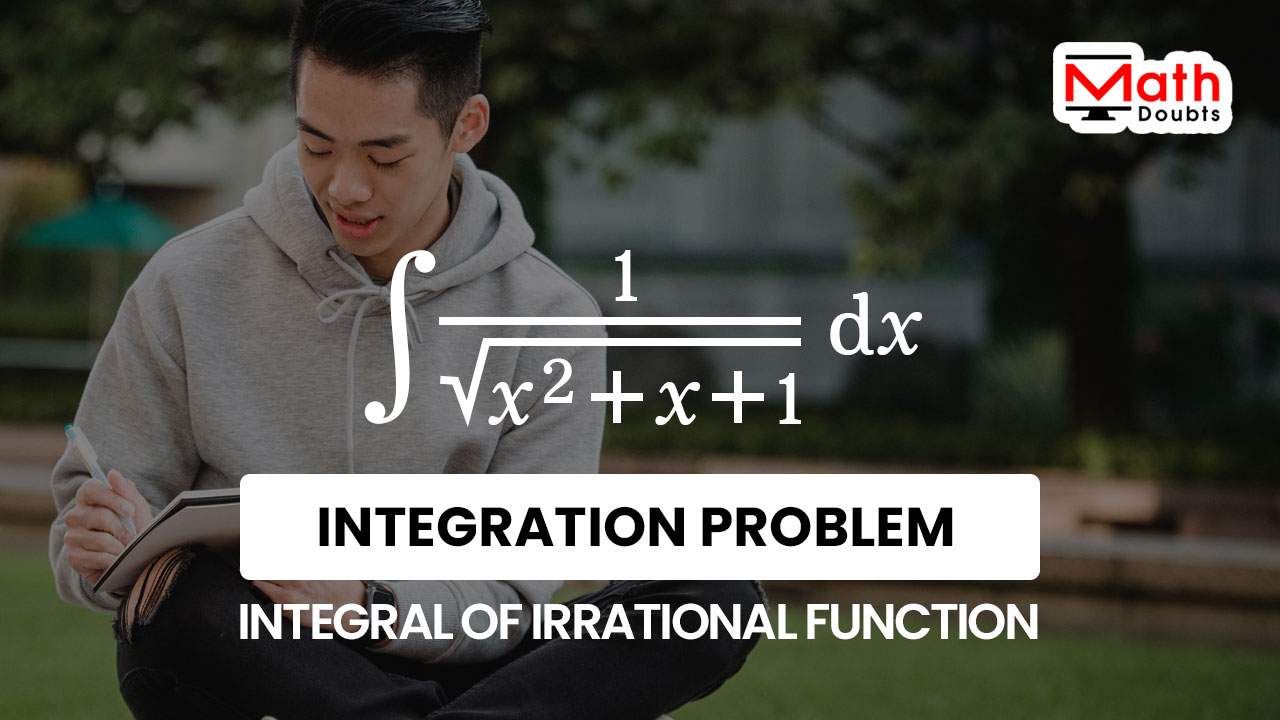
The reciprocal of the square root of $x$ square plus $x$ plus one is a rational function primarily but due to the involvement of the square root, it is also an irrational function. Now, let us learn how to find the indefinite integral of the multiplicative inverse of the square root of $x$ square plus $x$ plus one with respect to $x$.

Convert expression as sum of squares completely

The $x$ square plus $x$ plus one in the denominator under the square root is a second degree polynomial. It is tough to find the integral of the irrational function in reciprocal form but its indefinite integral can be calculated by completely converting the quadratic expression as the sum of two terms, with the help of the completing the square method.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+1 \times x+1}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+\dfrac{2}{2} \times x+1}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+\dfrac{2 \times 1}{2} \times x+1}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+2 \times \dfrac{1}{2} \times x+1}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+2 \times x \times \dfrac{1}{2}+1}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+2 \times x \times \dfrac{1}{2}+1+\bigg(\dfrac{1}{2}\bigg)^2-\bigg(\dfrac{1}{2}\bigg)^2}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{x^2+2 \times x \times \dfrac{1}{2}+\bigg(\dfrac{1}{2}\bigg)^2+1-\bigg(\dfrac{1}{2}\bigg)^2}}}\,dx$

The first three terms in the expression under the square root is the expansion of the square of sum of two terms rule. Hence, it can be simplified as the square of $x$ plus $1$ divided by $2$.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+1-\bigg(\dfrac{1}{2}\bigg)^2}}}\,dx$

The square of one divided by two belongs to both one and two, and the square form can be distributed to both numbers in the denominator under the square root by the power rule of a quotient.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+1-\dfrac{1^2}{2^2}}}}\,dx$

According to the exponentiation, the squares of one and two in the rational form can be evaluated.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+1-\dfrac{1}{4}}}}\,dx$

Now, it is time to subtract the one divided by four from the number one by the difference rule of the fractions.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\dfrac{4 \times 1-1 \times 1}{4}}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\dfrac{4-1}{4}}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\dfrac{3}{4}}}}\,dx$

The first term under the square root in the denominator is in square form. So, let us try to write $3$ divided by $4$ in square form by using the power of a quotient rule.

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\dfrac{\big(\sqrt{3}\big)^2}{2^2}}}}\,dx$

$=\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}}}\,dx$

Transform the function by the Change of variable

The quadratic expression under the square root in the denominator of a rational function is successfully converted as the sum of two squares but the binomial form in the first term does not allow us to use an integral rule in which the sum of two squares is there under square root in the denominator. However, it can be overcome by denoting the binomial $x$ plus one divided by two with another variable.

$y$ $\,=\,$ $x+\dfrac{1}{2}$

Now, differentiate the equation $y$ equals to $x$ plus one divided by $2$ with respect to $x$.

$\implies$ $\dfrac{d}{dx}\,y$ $\,=\,$ $\dfrac{d}{dx}\,\bigg(x+\dfrac{1}{2}\bigg)$

The derivative of variable $y$ with respect to $x$ is simply written as follows on the left hand side of the equation. On the right hand side of the equation, the derivative of sum of two terms $x$ and $1$ divided by $2$ should be evaluated. According to the sum rule of the integration, the differentiation should be distributed to both terms $x$ and one divided by two.

$\implies$ $\dfrac{dy}{dx}$ $\,=\,$ $\dfrac{d}{dx}\,(x)$ $+$ $\dfrac{d}{dx}\,\bigg(\dfrac{1}{2}\bigg)$

The derivative of $x$ with respect to $x$ is equal to $1$, as per the derivative rule of a variable. According to the derivative rule of a constant, the derivative of $1$ divided by $2$ with respect to $x$ is zero.

$\implies$ $\dfrac{dy}{dx}$ $\,=\,$ $1$ $+$ $0$

It is now time to find the value of the differential element in a variable $y$ by simplifying the equation.

$\implies$ $\dfrac{dy}{dx}$ $\,=\,$ $1$

$\implies$ $dy$ $\,=\,$ $1 \times dx$

$\,\,\,\therefore\,\,\,\,\,\,$ $dy$ $\,=\,$ $dx$

The derivative of the reciprocal of an irrational function in terms of $x$ can be converted as the derivative of an irrational function in $y$ by changing the variable $x$.

$\,\,\,\therefore\,\,\,\,\,\,$ $\displaystyle \int{\dfrac{1}{\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}}}\,dx$ $\,=\,$ $\displaystyle \int{\dfrac{1}{\sqrt{y^2+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}}}\,dy$

Find the integral of the function in irrational form

The quadratic expression is now successfully converted as the sum of two terms. Now, the integral of, the reciprocal of, the square root of $y$ square plus square of square root of three divided by two with respect to $y$ can be calculated by an integral rule of an irrational function in reciprocal form.

$=\,\,\,$ $\log_{e}{\Bigg|y+\sqrt{y^2+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}\Bigg|}$ $+$ $c$

We have considered that the value a variable y is equal to $x$ plus half. So, replace the value of variable $y$ by its value in terms of $x$ in the above logarithmic expression.

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{\bigg(x+\dfrac{1}{2}\bigg)^2+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}\Bigg|}$ $+$ $c$

Now, simplify the algebraic expression inside the natural logarithmic function and under the square root to get the integral in simplified form. The square of sum can be expanded by the square rule of the sum.

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+\bigg(\dfrac{1}{2}\bigg)^2+2 \times x \times \dfrac{1}{2}+\bigg(\dfrac{\sqrt{3}}{2}\bigg)^2}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+\dfrac{1^2}{2^2}+\dfrac{2 \times x \times 1}{2}+\dfrac{\big(\sqrt{3}\big)^2}{2^2}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+\dfrac{1}{4}+\dfrac{2 \times x}{2}+\dfrac{3}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+\dfrac{1}{4}+\dfrac{\cancel{2} \times x}{\cancel{2}}+\dfrac{3}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+\dfrac{1}{4}+x+\dfrac{3}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+x+\dfrac{1}{4}+\dfrac{3}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+x+\dfrac{1+3}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+x+\dfrac{4}{4}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+x+\dfrac{\cancel{4}}{\cancel{4}}}\Bigg|}$ $+$ $c$

$=\,\,\,$ $\log_{e}{\Bigg|\bigg(x+\dfrac{1}{2}\bigg)+\sqrt{x^2+x+1}\Bigg|}$ $+$ $c$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.