The interior angles which appear opposite sides of transversal of nonparallel lines are called the alternate interior angles formed by nonparallel lines and their transversal line.
When two nonparallel lines are intersected by their transversal, four interior angles are formed geometrically but every two of them are appeared opposite sides at the intersection of the non-parallel lines by their transversal line. Hence, two pairs of alternate interior angles are formed by the intersection.
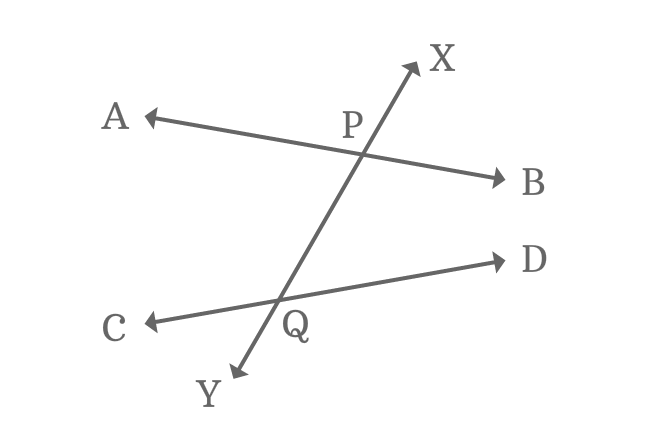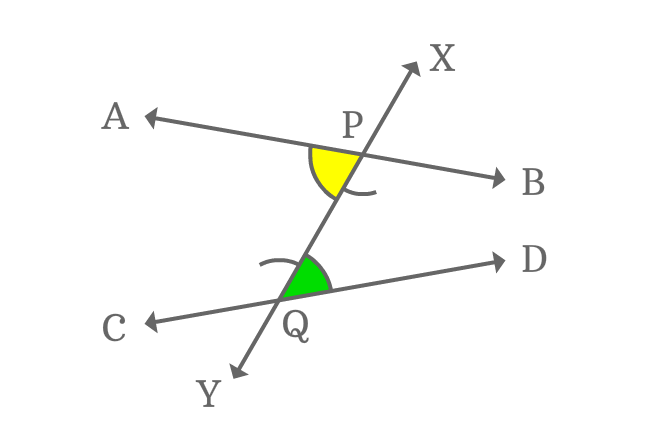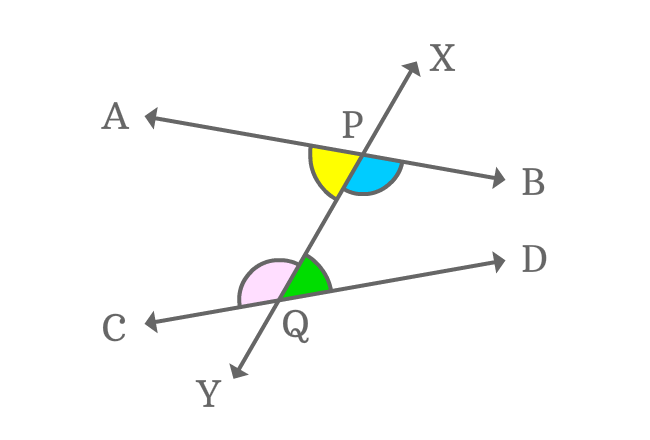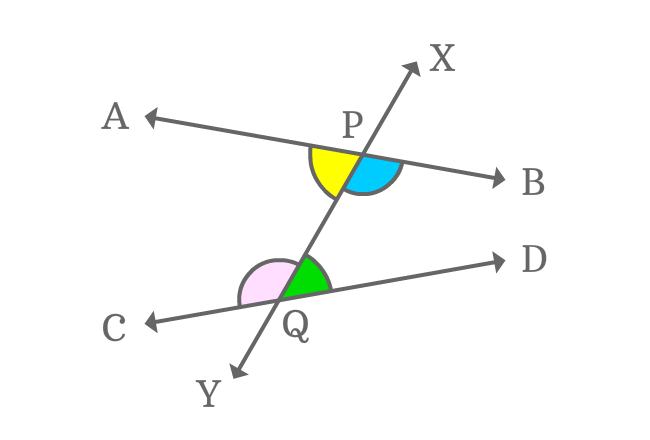
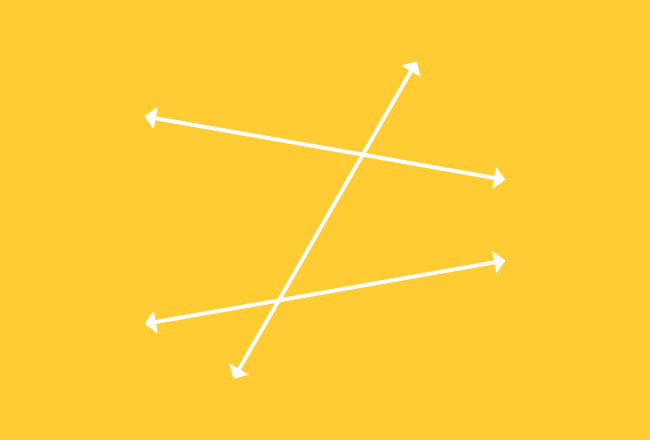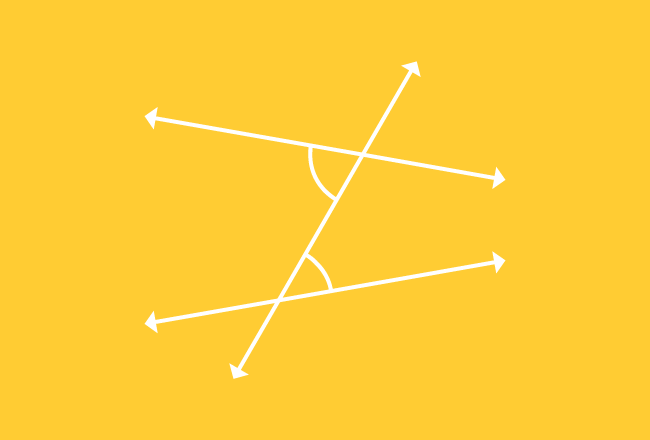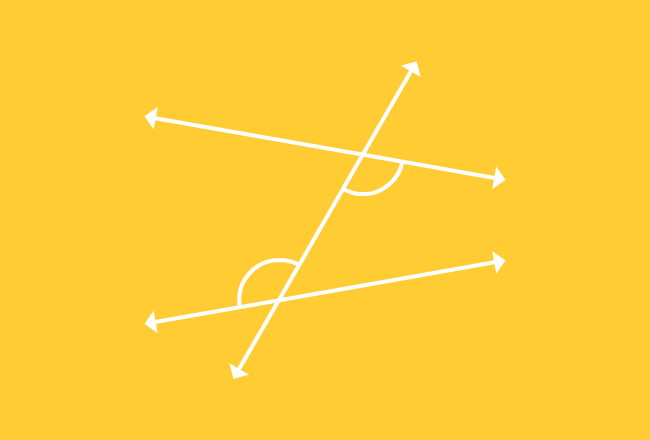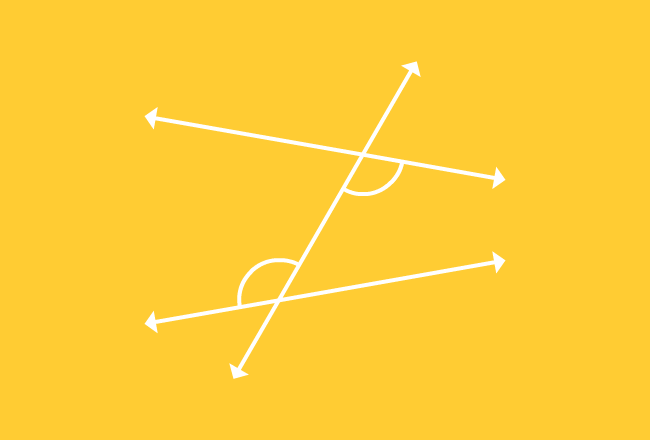
When two nonparallel lines ($\overleftrightarrow{AB}$ and $\overleftrightarrow{CD}$) are intersected by their transversal line $\overleftrightarrow{XY}$, four interior angles $\angle YPA$, $\angle YPB$, $\angle XQC$ and $\angle XQD$ are formed geometrically.
The following two pairs of interior angles are alternate interior angles.
$1. \,\,\,\,\,\,$ $\angle YPA$ and $\angle XQD$
$2. \,\,\,\,\,\,$ $\angle YPB$ and $\angle XQC$
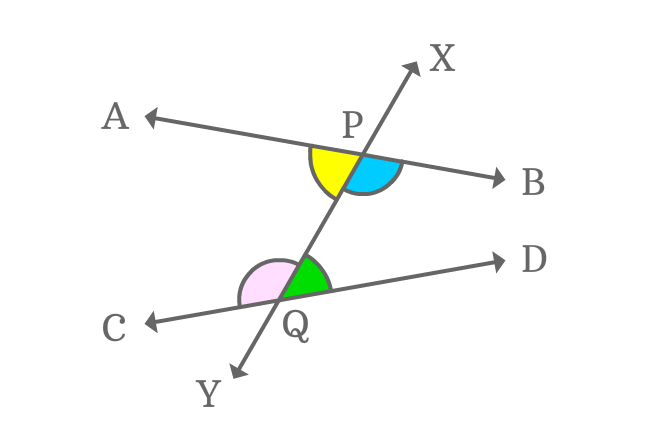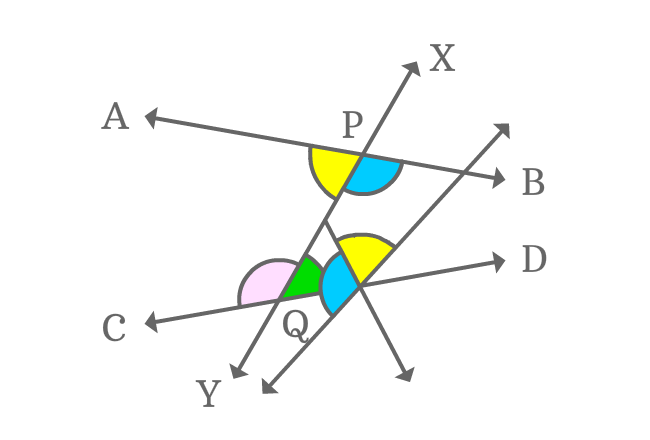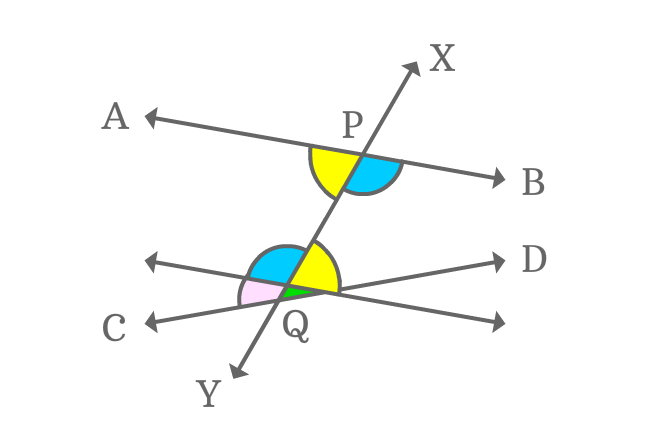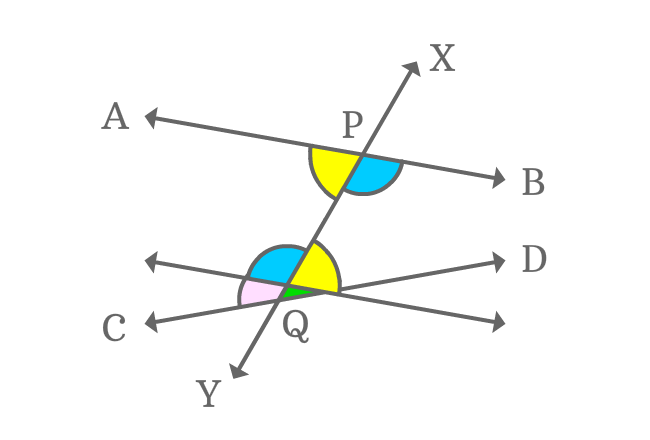
Each pair of alternate interior angles are not equal. It is mainly due to the involvement of nonparallel lines in the intersection with their transversal line.
$1. \,\,\,\,\,\,$ $\angle YPA \,\ne\, \angle XQD$
$2. \,\,\,\,\,\,$ $\angle YPB \,\ne\, \angle XQC$
A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.
Copyright © 2012 - 2023 Math Doubts, All Rights Reserved