Cosine angle difference identity

Formula

$(1).\,\,$ $\cos{(a-b)}$ $\,=\,$ $\cos{a}\cos{b}$ $+$ $\sin{a}\sin{b}$

$(2).\,\,$ $\cos{(x-y)}$ $\,=\,$ $\cos{x}\cos{y}$ $+$ $\sin{x}\sin{y}$

Introduction

Let $a$ and $b$ be two variables, which are used to represent two angles in this case. The subtraction of angle $b$ from angle $a$ is the difference between them, and it is written as $a-b$, which is a compound angle. The cosine of a compound angle $a$ minus $b$ is written as $\cos{(a-b)}$ in trigonometry.

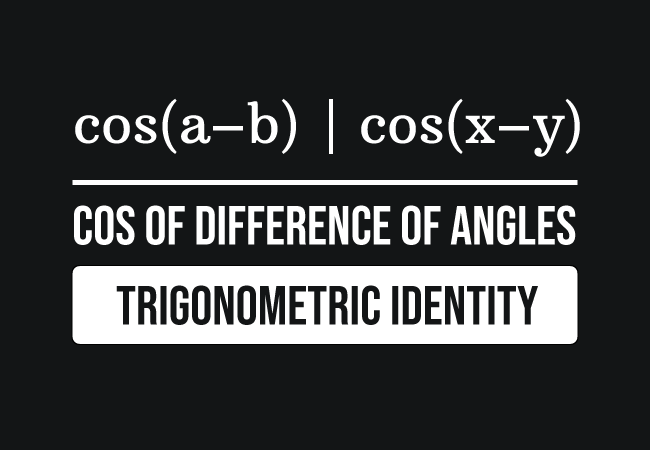
The cosine of subtraction of angle $b$ from angle $a$ is equal to the sum of the products of cosines of angles $a$ and $b$, and sines of angles $a$ and $b$.

$\cos{(a-b)}$ $\,=\,$ $\cos{a} \times \cos{b}$ $+$ $\sin{a} \times \sin{b}$

This mathematical equation can be called the cosine angle difference trigonometric identity in mathematics.

Usage

The cosine angle difference identity is possibly used in two different cases in trigonometry.

Expansion

The cosine of difference of two angles is expanded as the sum of the products of cosines of angles and sines of angles.

$\implies$ $\cos{(a-b)}$ $\,=\,$ $\cos{(a)}\cos{(b)}$ $+$ $\sin{(a)}\sin{(b)}$

Simplification

The sum of the products of cosines of angles and sines of angles is simplified as the cosine of difference of two angles.

$\implies$ $\cos{(a)}\cos{(b)}$ $+$ $\sin{(a)}\sin{(b)}$ $\,=\,$ $\cos{(a-b)}$

Forms

The angle difference identity in cosine function is written in several forms but the following three forms are some popularly used forms in the world.

$(1).\,\,$ $\cos{(A-B)}$ $\,=\,$ $\cos{A}\cos{B}$ $+$ $\sin{A}\sin{B}$

$(2).\,\,$ $\cos{(x-y)}$ $\,=\,$ $\cos{x}\cos{y}$ $+$ $\sin{x}\sin{y}$

$(3).\,\,$ $\cos{(\alpha-\beta)}$ $\,=\,$ $\cos{\alpha}\cos{\beta}$ $+$ $\sin{\alpha}\sin{\beta}$

Proof

Learn how to derive the cosine of angle difference trigonometric identity by a geometric method in trigonometry.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.