Formation of an Angle by the Rotation

Definition

The formation of an angle by rotating a straight line with a fixed endpoint on a plane is called the formation of an angle by rotation.

Introduction

Geometrically, an angle is formed, when a straight line with a fixed endpoint is rotated to another position from its initial position on a plane. So, it is called the formation of an angle by rotation.

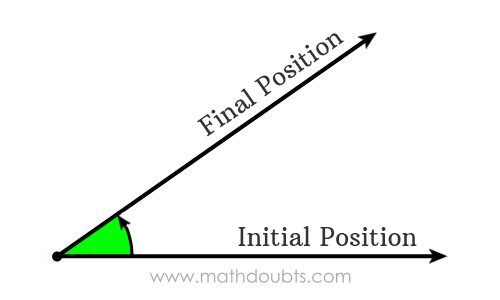
It is a fundamental geometric theory to explain the formation of an angle by rotating a straight line. Now, let’s learn how an angle is formed by rotation from an understandable graphical animation.

Example

Firstly, let’s draw a geometric figure by the below steps.

1. Consider a point and let’s denote it by $O$ on a plane.
2. Draw a straight line from that point. Now, rotate the straight line with its fixed starting point.
3. Let’s assume that $P$ is a point on the straight line, when it is in initial position and $Q$ is a point on the straight line, when it is in final position.

The ray $\overrightarrow{OP}$ becomes $\overrightarrow{OQ}$ when the ray $\overrightarrow{OP}$ is rotated from its initial position. Now, an $angle \, POQ$ is formed between the rays $\overrightarrow{OP}$ and $\overrightarrow{OQ}$.

In this way, an angle Is formed geometrically, when a straight line is rotated to a position from its initial position. There is also another theory to know the formation of an angle. Now, let’s learn how an angle is formed between two lines.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.