A triangle whose lengths of all three sides are equal is called an equilateral triangle.
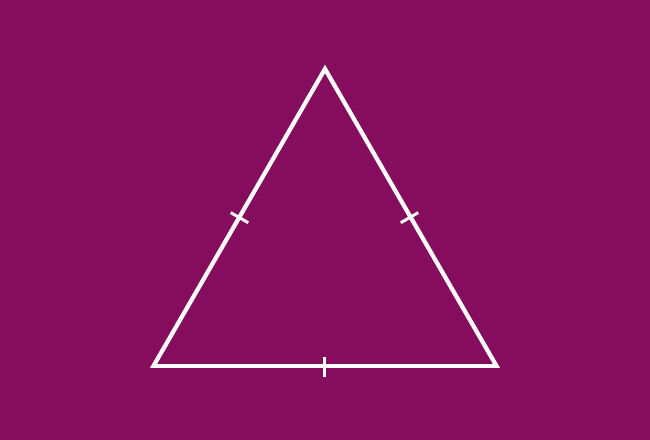
A triangle can be constructed by connecting endpoints of three line segments of same length as a closed geometric shape. Actually, the meaning of equilateral is sides of the same length. So, the triangle is called as an equilateral triangle.
The lengths of all three sides of an equilateral triangle are equal due to the construction of triangle with the line segments which have same length. Due to same reason, its interior angles are also equal and each angle is equal to $60^°$. Hence, it is also called as an equiangular triangle.
$\Delta EFG$ is a triangle and length of each side is $l$. So, this triangle is called as an equilateral triangle.
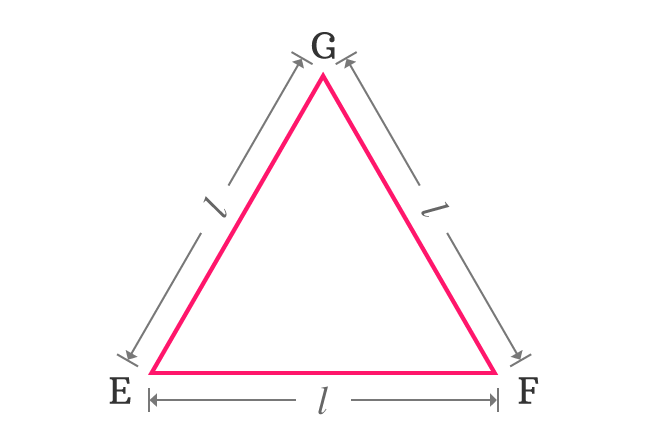
The lengths of all three sides of $\Delta EFG$ are equal.
$EF = FG = GE = l$
It is an equiangular triangle. So, all three angles of this triangle are equal and each angle is $60^°$.
$\angle EFG$ $\,=\,$ $\angle FGE$ $\,=\,$ $\angle GEF$ $\,=\,$ $60^°$
Learn how to construct an equilateral triangle geometrically by using geometric tools.
A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.
Copyright © 2012 - 2025 Math Doubts, All Rights Reserved