Evaluate $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\sqrt{1+x}-1}{x}}$ by Rationalization

The limit of square root of one plus $x$ minus one divided by $x$ is indeterminate as the value of $x$ approaches zero as per the direct substitution method.

$\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\sqrt{1+x}-1}{x}}$ $\,=\,$ $\dfrac{0}{0}$

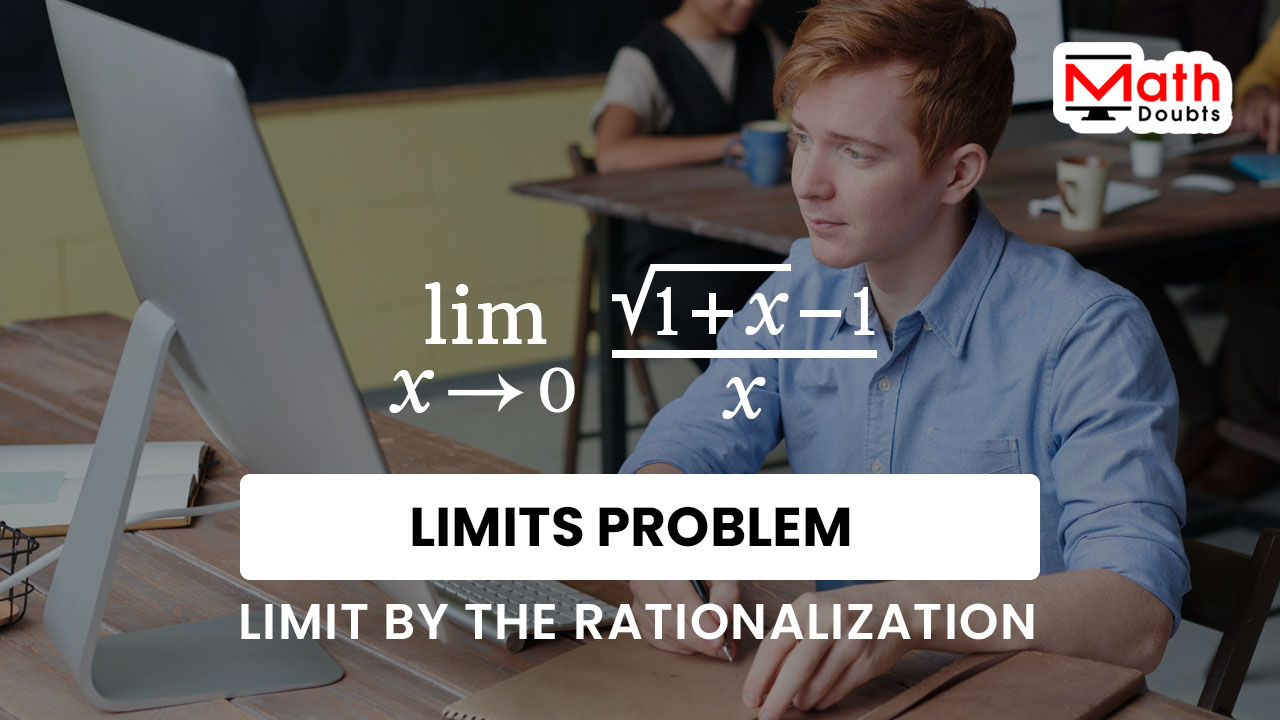
It is given in this limit question that the limit of square root of $1$ plus $x$ minus $1$ divided by $x$ should be evaluated by rationalization as the value of $x$ tends to $0$.

Remove the indeterminate form by Rationalisation

An expression in radical form the square root of $1$ plus $x$ is involved in forming the function in the numerator. So, let us try to remove the irrational form of the expression in the numerator by rationalizing it with its conjugate function.

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \bigg(\dfrac{\sqrt{1+x}-1}{x}}$ $\times$ $1\bigg)$

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \bigg(\dfrac{\sqrt{1+x}-1}{x}}$ $\times$ $\dfrac{\sqrt{1+x}+1}{\sqrt{1+x}+1}\bigg)$

Find the Product by simplifying Rational function

Now, it is time to multiply the two rational expressions consisting irrational form expressions by the multiplication rule of fractions.

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\big(\sqrt{1+x}-1\big) \times \big(\sqrt{1+x}+1\big)}{x \times \big(\sqrt{1+x}+1\big)}}$

The product of the functions in the numerator can be multiplied by the difference of squares formula.

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\big(\sqrt{1+x}\big)^2-1^2}{x\big(\sqrt{1+x}+1\big)}}$

Now, let us focus on simplifying the expressions in both numerator and denominator of the rational function.

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{1+x-1}{x\big(\sqrt{1+x}+1\big)}}$

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\cancel{1}+x-\cancel{1}}{x\big(\sqrt{1+x}+1\big)}}$

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{x}{x\big(\sqrt{1+x}+1\big)}}$

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{\cancel{x}}{\cancel{x}\big(\sqrt{1+x}+1\big)}}$

$=\,\,$ $\displaystyle \large \lim_{x\,\to\,0}{\normalsize \dfrac{1}{\sqrt{1+x}+1}}$

Evaluate the Limit by Direct substitution

Now, let us find the limit of the reciprocal of square root of one plus $x$ plus one by substituting $x$ is equal to zero directly.

$=\,\,$ $\dfrac{1}{\sqrt{1+0}+1}$

$=\,\,$ $\dfrac{1}{\sqrt{1}+1}$

$=\,\,$ $\dfrac{1}{1+1}$

$=\,\,$ $\dfrac{1}{2}$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.