Evaluate $\dfrac{d}{dx}\sqrt{1+x}$ by the Chain rule in Fundamental notation

The derivative of the square root of $1$ plus $x$ with respect to $x$ should be evaluated in this differentiation question by using the chain rule. The $\sqrt{1+x}$ is an irrational function in algebraic form. In differential calculus, there is no differentiation formula to find the derivative of the square root of $1$ plus $x$.

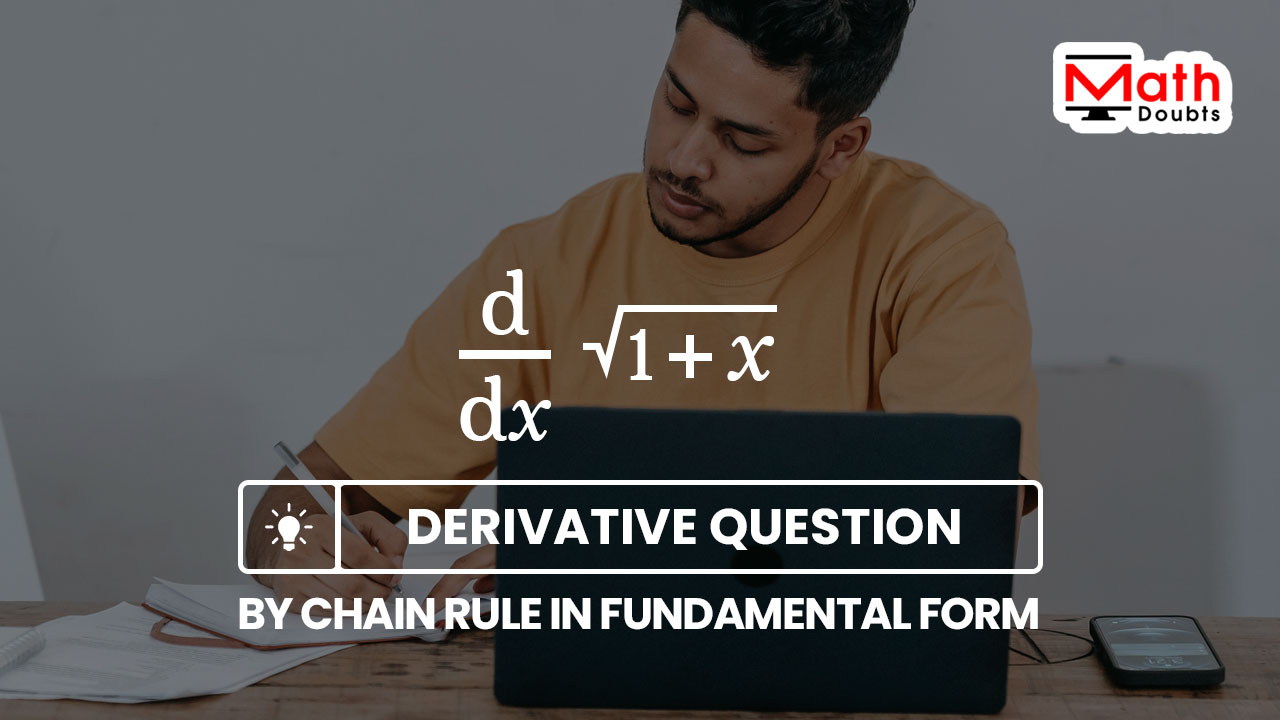
However, it can be evaluated by the chain rule. Let’s learn how to find the derivative of the square root of $1$ plus $x$ with respect to $x$ by the chain rule in fundamental notation.

Express the Derivative of function by Chain Rule

The $\sqrt{1+x}$ function can be considered as the composition of two functions $\sqrt{x}$ and $1+x$. So, the derivative of composite function $\sqrt{1+x}$ can be evaluated now with respect of $x$ by using the chain rule in fundamental notation.

$\dfrac{d}{dx}f\big(g(x)\big)$ $\,=\,$ $\dfrac{d}{d\,g(x)}f\big(g(x)\big)$ $\times$ $\dfrac{d}{dx}g(x)$

Let’s take $f\big(g(x)\big)$ $\,=\,$ $\sqrt{1+x}$, then $g(x)$ $\,=\,$ $1+x$. Now, substitute them in the chain rule to start the process of differentiation of the square root of $1+x$ with respect to $x$.

$\implies$ $\dfrac{d}{dx}\sqrt{1+x}$ $\,=\,$ $\dfrac{d}{d(1+x)}\sqrt{1+x}$ $\times$ $\dfrac{d}{dx}(1+x)$

Find the derivatives of functions in the expression

Let’s find the derivative of the square root of $1$ plus $x$ with respect to $x$ by finding the product of the derivative of the square root of $1$ plus $x$ with respect to $1$ plus $x$ and the derivative of $1$ plus $x$ with respect to $x$.

$=\,\,$ $\dfrac{d}{d(1+x)}\sqrt{1+x}$ $\times$ $\dfrac{d}{dx}(1+x)$

Firstly, let’s find the derivative of the square root of $1$ plus $x$ with respect to $1$ plus $x$. It may actually confuse you. So, denote the $1+x$ by a variable $y$ but keep the second factor as it is.

$=\,\,$ $\dfrac{d}{d(y)}\sqrt{y}$ $\times$ $\dfrac{d}{dx}(1+x)$

$=\,\,$ $\dfrac{d}{dy}\sqrt{y}$ $\times$ $\dfrac{d}{dx}(1+x)$

The derivative of the square root of $y$ with respect to $y$ can be evaluated by the derivative rule of a square root function. So, the derivative of square root of $y$ with respect to $y$ is equal to the reciprocal of two times square root of $y$.

$=\,\,$ $\dfrac{1}{2\sqrt{y}}$ $\times$ $\dfrac{d}{dx}(1+x)$

The variable $y$ is considered to represent the algebraic function $1+x$. Now, replace the variable $y$ by its actual value in $x$ in the first factor of the expression.

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$ $\times$ $\dfrac{d}{dx}(1+x)$

It is time to find the derivative of an algebraic function $1$ plus $x$. The algebraic function $1$ plus $x$ is formed by addition of two functions. So, the differentiation of sum of two functions $1$ and $x$ can be evaluated by the sum rule of the derivatives.

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$ $\times$ $\bigg(\dfrac{d}{dx}\,1$ $+$ $\dfrac{d}{dx}\,x\bigg)$

The derivative of one with respect to $x$ is zero as per the derivative rule of a constant, and the derivative of $x$ with respect to $x$ is equal to one as per the derivative rule of a variable.

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$ $\times$ $(0+1)$

Evaluate the Derivative of function by simplification

The derivative of $1$ plus $x$ with respect to $x$ is successfully evaluated as an expression. So, let’s simplify the expression to find the differentiation of $1$ plus $x$ with respect to $x$ by the chain rule.

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$ $\times$ $(1)$

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$ $\times$ $1$

The first factor is an irrational function in fraction form and the second factor is a natural number. The product of them can be evaluated by the multiplication rule of the fractions.

$=\,\,$ $\dfrac{1 \times 1}{2\sqrt{1+x}}$

$=\,\,$ $\dfrac{1}{2\sqrt{1+x}}$

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.