$A \,=\, 2lw+\pi(R^2-r^2)$
The area of running track can be expressed in mathematical form and it can be used as a formula in mathematics to find the area of any running track field. It can be derived easily in mathematical form when you understand the geometrical science behind the construction of a running track.

Geometrically, the shape of a running track is the combination of both rectangle and annulus. So, you first have to learn how to find the area of a rectangle and the area of a circular ring.
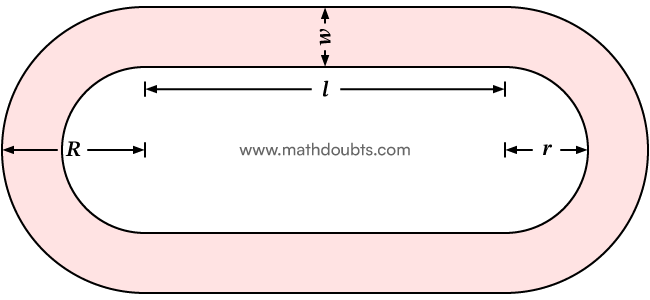
Now, let’s denote the various dimensions of running track field algebraically as follows.
The area of a running track is equal to the sum of two times the area of rectangular lane and the area of annulus lane.
$A \,=\, 2lw+\pi(R^2-r^2)$
Learn how to derive a formula in algebraic form to find the area of a running track in mathematics.
A worksheet of list of math questions on finding the areas of running track fields for your practice with solutions to learn how to find the area of any running track mathematically.
A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.
Copyright © 2012 - 2025 Math Doubts, All Rights Reserved