Csc of negative angle

A mathematical relation of Cosecant of negative angle with Cosecant of positive angle is called Cosecant of negative angle identity.

Proof

The mathematical relation between Cosecant of positive angle and Cosecant of negative angle is derived mathematically in trigonometry by geometrical method.

Construction of triangle with positive angle

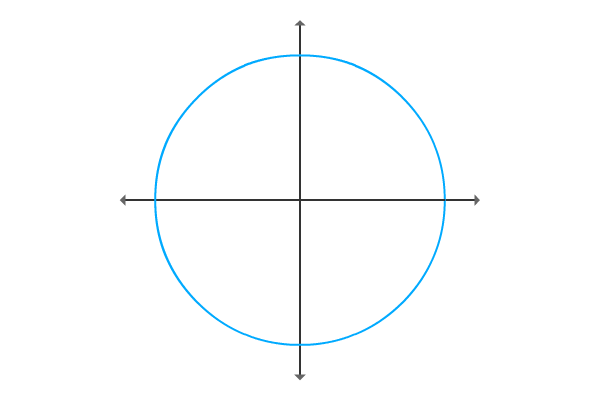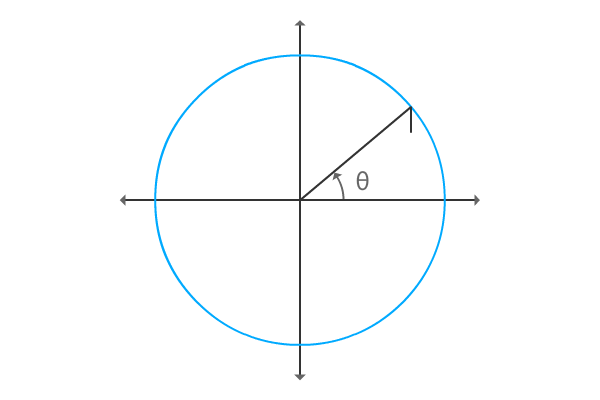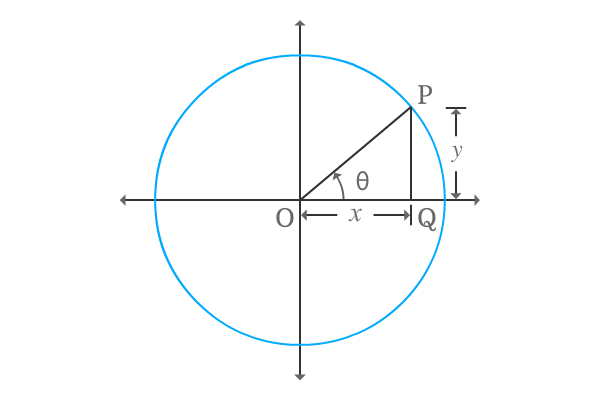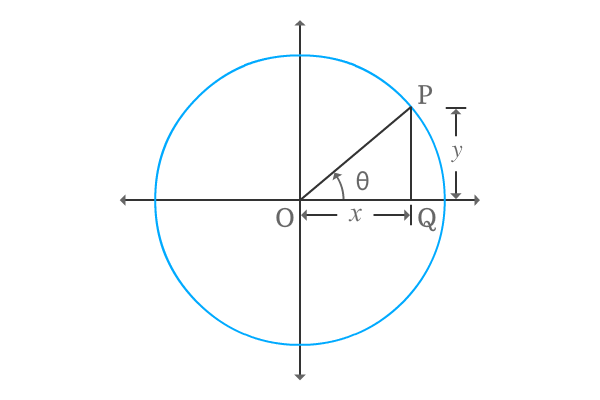
$\Delta POQ$ is a right angled triangle and its construction is done geometrically with a positive angle theta. So, express cosecant of positive angle in terms of ratio of lengths of the corresponding sides.

$\csc{\theta} \,=\, \dfrac{OP}{PQ}$

However, this triangle is construction in first quadrant. Therefore, the lengths of both adjacent and opposite sides are positive and denoted by $x$ and $y$ respectively.

$\implies$ $\csc{\theta} \,=\, \dfrac{\sqrt{x^2+y^2}}{y}$

Construction of triangle with negative angle

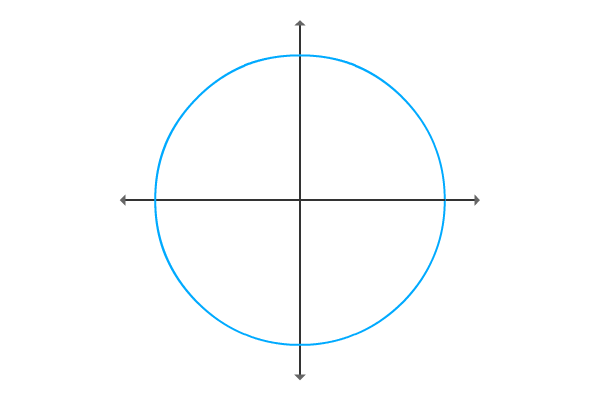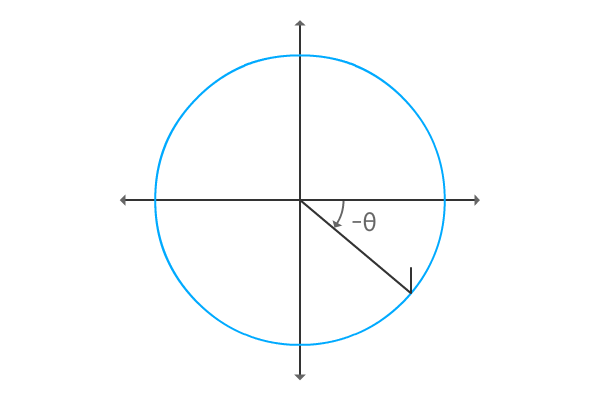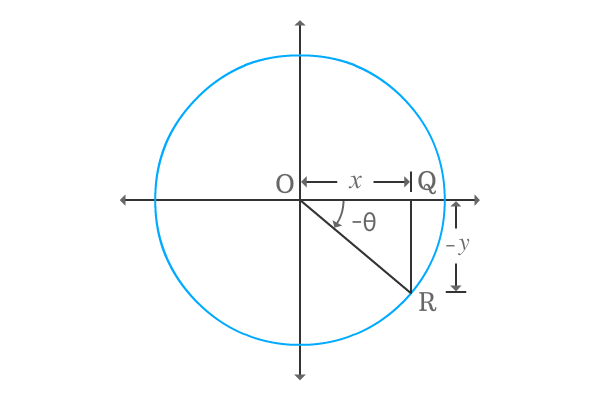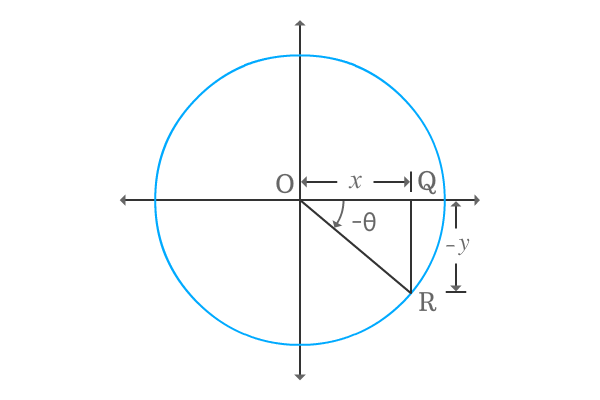
Similarly, construct same triangle with negative angle but the magnitude of this triangle should be same. Therefore, the angle of $\Delta ROQ$ is negative theta, represented by $–\theta$.

Now, express the trigonometric ratio co-secant in terms of ratio of the lengths of associated sides.

$\csc{(-\theta)} \,=\, \dfrac{OR}{RQ}$

The length of opposite side will be negative because of construction of triangle with negative angle. Geometrically, the length of the opposite side will be $–y$ but the length of adjacent side does not chance.

$\implies$ $\csc{(-\theta)} \,=\, \dfrac{\sqrt{x^2+y^2}}{-y}$

$\implies$ $\csc{(-\theta)} \,=\, -\dfrac{\sqrt{x^2+y^2}}{y}$

Comparing sine functions

Now, investigate the relation between cosecant of positive angle and cosecant of negative angle by comparing them.

$\csc{\theta} \,=\, \dfrac{\sqrt{x^2+y^2}}{y}$

$\csc{(-\theta)} \,=\, -\dfrac{\sqrt{x^2+y^2}}{y}$

The comparison of both cosecant functions disclose that cosecant of negative angle equals to negative of cosecant of positive angle.

$\csc{(-\theta)} \,=\, -(\csc{\theta})$

$\,\,\, \therefore \,\,\,\,\,\,$ $\csc{(-\theta)} \,=\, -\csc{\theta}$

This negative identity is called cosecant of negative angle identity and frequently used as a formula in trigonometric mathematics.

A free math education service for students to learn every math concept easily, for teachers to teach mathematics understandably and for mathematicians to share their maths researching projects.